Bike Crank Analysis
Displacement, deformation, and stress analysis on a bike crank
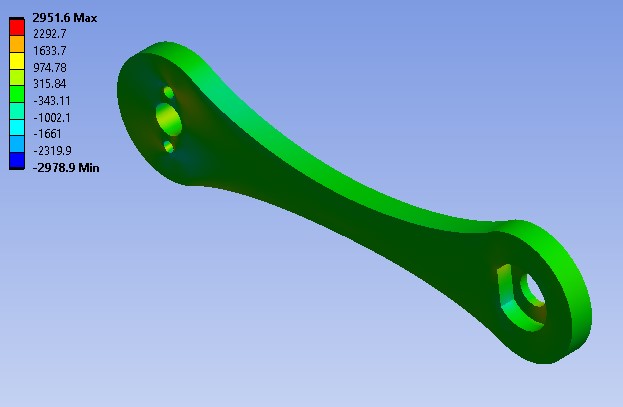
The bicycle crank is a component of a bicycle that connects the pedals to the bicycle frame. In this project, the bike crank was modeled and analyzed using ANSYS. Results such as total deformation and stress distribution of the crank are shown below.
Mesh & Setup
X-Y plane mesh
Isometric mesh
Isometric forces
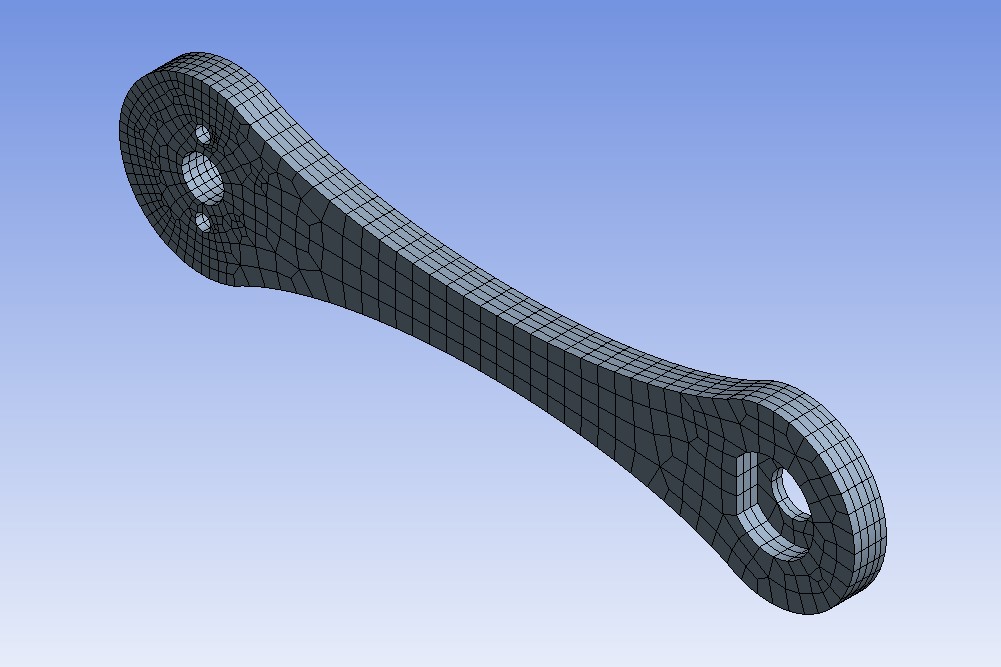
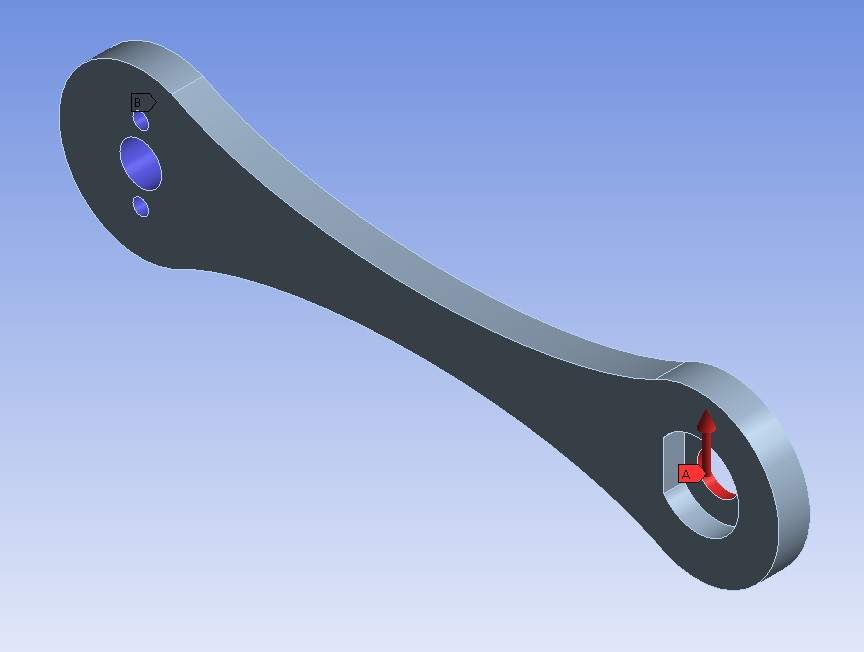
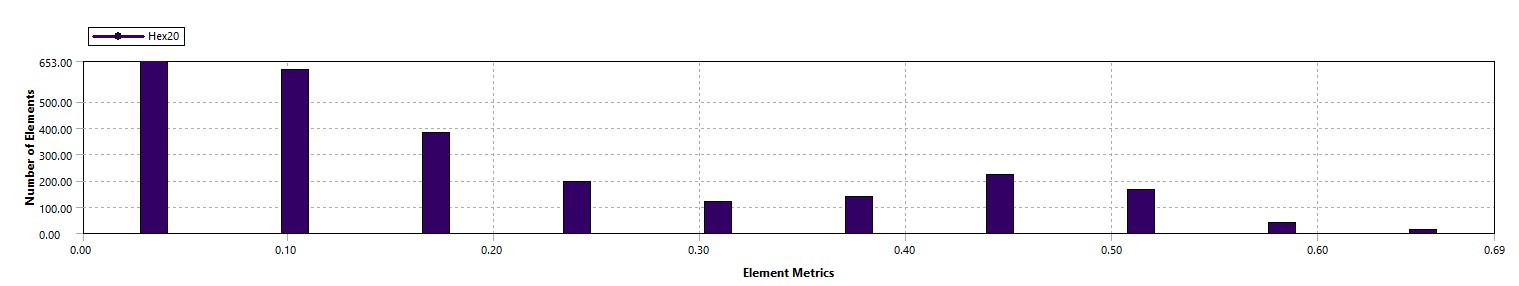
When generating the bike crank's mesh, focus was placed on the holes at each side of the crank with coarser meshing at the middle of the bar. The model in this project is simplified by changing the variable force that a rider usually exerts on the crank to a static force of 100lbf. The material used in this analysis is aluminum 6061-76 alloy. In addition to the 100lbf added to one side of the crank, a fixed boundary condition was set to the opposite. This fixed position sets up the crank to be modeled almost as a cantilever beam with the 100lbf side being expected to have the max deformation once the solution is generated.
Results
Deformation
X-axis normal stress
Y-axis normal stress
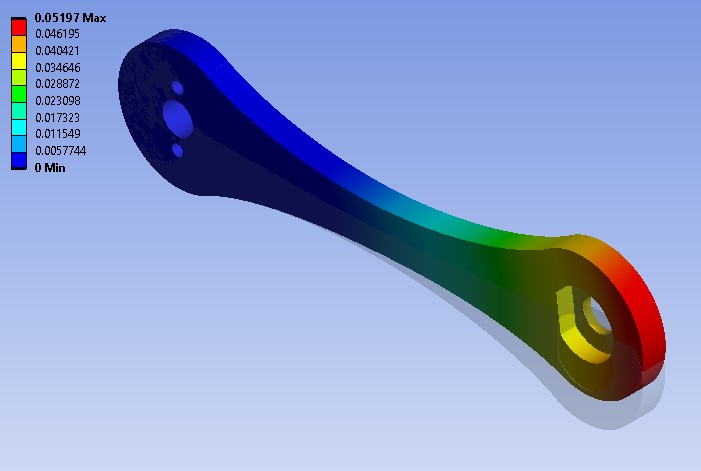
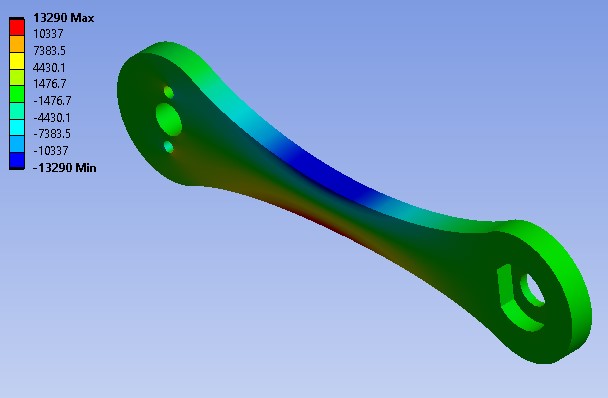
The max deformation seen in the bike crank was around 0.05 inches at the side with the applied force which is what was expected. Since the opposite side was fixed it also makes sense that there would be close to no deflection there. For the X-axis normal stress we see that there is an equal stress at the top and bottom of the midsection of around 13ksi. This is due to the bike crank being in tension at the bottom and compression at the top. When looking at the Y-axis normal stress we see that the values are significantly lower especially in the middle where it is close to 0. This is due to the reaction force of 100lbf being set in that axis.
Error Analysis
Orthogonal quality
Skewness
As shown above, the mesh quality was checked in order to make sure the output was accurate. The mesh's average orthogonal quality and skewness both had great values at 0.956 and 0.196, respectively. Perfect values for these would be an orthogonal quality of 1 and a skewness of 0 which were close in this case. Aside from the mesh, there is error due to simplification of the model such as not accounting for gravity, using a static force approximation, And using a simple geometry. If this analysis had to be refined further for a precise answer to the deformation and stress values, these variables should be accounted for.